Talk:Tusi couple

	History of science portal This article is part of the History of Science WikiProject, an attempt to improve and organize the history of science content on Wikipedia. If you would like to participate, you can edit the article attached to this page, or visit the project page, where you can join the project and/or contribute to the discussion. You can also help with the History of Science Collaboration of the Month.History of ScienceWikipedia:WikiProject History of ScienceTemplate:WikiProject History of Sciencehistory of science articles
Low	This article has been rated as Low-importance on the project's importance scale.

Middle Ages Low‑importance

	Middle Ages portal This article is within the scope of WikiProject Middle Ages, a collaborative effort to improve the coverage of the Middle Ages on Wikipedia. If you would like to participate, please visit the project page, where you can join the discussion and see a list of open tasks.Middle AgesWikipedia:WikiProject Middle AgesTemplate:WikiProject Middle AgesMiddle Ages articles
Low	This article has been rated as Low-importance on the project's importance scale.

Incorrect Math

The statement "A property of the Tusi couple is that points on the inner circle that are not on the circumference trace ellipses" is not correctly stated. There can not be any points on the inner circle that are not on its circumference, because, by construction, all points on a circle lie on the circumference of that circle. What is meant would be correctly expressed if we are talking about all points of a disk that is co-rotating with the inner circle. Only then is it true that there can be points of the disk not on the circumference of the circle, and that those points not on the circle's circumference trace ellipses. Suggested replacement would be:

"A property of the Tusi couple is that all points of a disk of any radius co-rotating with the inner circle trace out ellipses. Points on the circumference of the circle or at the center of the circle trace out degenerate ellipses (straight line and circle, respectively.)"

173.243.178.98 (talk) 20:44, 24 January 2020 (UTC)[reply]

Peer Review

Dear Duythai25,

I like the division of information and everything looks fleshed out now. Maybe you could add more about epicycles and how Western theories using them were different than Arab counterparts. SebCoyle (talk) 16:33, 16 April 2014 (UTC)[reply]

Copyright violation?

The opening lines "The Tusi-couple ... (Sotiroudis and Paschos 1999, p. 60; Kanas 2003)" are taken verbatim from [1], without citation (and without giving the full form of the references cited there).

Description of Tusi Couple

I have removed Maestlin's sentence "The large circle rotates in the opposite direction at half the speed, carrying the small circle with it." since the two animations of the Tusi Couple in the external links both have the small circle rotating within a fixed outer circle. There is also a way to depict the Tusi couple in which two circles of the same size rotate in opposite directions, but this is not the one in the animations or in the diagram from the Vatican manuscript of Tusi's invention. Di Bono's article in JHA discusses the various ways to depict the Tusi couple.

--SteveMcCluskey 00:14, 20 June 2006 (UTC)[reply]

I will edit the article to incorporate what Tusi said about the rectilinear Tusi couple, since accuracy needs to take precedence over keeping the article consistent with external links. F.J. Ragep addresses the "rolling" question in his commentary on Tusi's Memoir on Astronomy:

"In point of fact, Tusi does not need two motions to achieve the oscillation of his given point along the diameter of the larger circle; he merely needs to allow the smaller circle to "roll" inside the larger one, which would remain stationary. ... I have belabored this rather obvious point in order to underscore the fact that Tusi does not proceed in this manner. The reason is that this lemma is not simply a mathematical theorem; it is meant to have a physical application and therefore the added stipulations are necessary. For there can be no rolling in the heavens; only rotation in place is allowed since there is no void. It is unfortunate that Kennedy--and others--have persisted in calling the Tusi couple a "rolling device," which is emphatically is not." (vol. 2, pp. 433-34)

If you think that the other two versions of the Tusi couple should be included, don't let the lack of animations or diagrams stop you from writing up a description. Maestlin 18:48, 20 June 2006 (UTC)[reply]

Oh dear

Well, here we go again. Its an Arabic thing... but actually appears in commentaries on Euclid more than 500 years earlier. Major rephrasing is required. I suspect the hand of Jagged William M. Connolley (talk) 20:05, 21 April 2011 (UTC)[reply]

Funny you say that, given that this was the only change committed by Jagged85 to this article. Bias ? Al-Andalusi (talk) 20:48, 21 April 2011 (UTC)[reply]

Pardon? William M. Connolley (talk) 21:07, 21 April 2011 (UTC)[reply]

Anyway, there is no source for It was developed by the 13th-century Persian astronomer and mathematician Nasir al-Din al-Tusi as an alternative to the problematic equant. Who says it is an alternative to the equant? William M. Connolley (talk) 21:20, 21 April 2011 (UTC)[reply]

E. S. Kennedy. He gives an explanation of the origin of the Tusi couple in his paper "Late Medieval Planetary Theory", Isis 57, #3 (Autumn 1966), 365-378, JSTOR 228366. According to him, Nasir al-Din al-Tusi was dissatisfied with the equant, which, as you'll recall, involved making the center of the epicycle travel around a deferent circle, while its motion around this circle was uniform with respect to not the center of the deferent, but a different center (the equant point.) This was philosophically unsatisfactory as it violated the Aristotelean principle of uniform circular motion. The solution al-Tusi came up with was to start with the equant point and have a point uniformly moving around that in a circle. Then he stuck a Tusi couple on top of this point and made its endpoint the center of the epicycle. The result is a path which is quite close to the Ptolemaic path. There is an animation of al-Tusi's model, along with some other models, here (another version here; main page here.) So, the statement is basically correct, although it's imprecise in that what avoids the equant is Tusi's overall planetary model, of which the couple is only one piece. Spacepotato (talk) 00:48, 22 April 2011 (UTC)[reply]

Thanks. I was dubious because, if I recall correctly, Copernicus uses it for something totally different (some equinox wobble that turned out not to really exist, I think) William M. Connolley (talk) 08:40, 22 April 2011 (UTC)[reply]

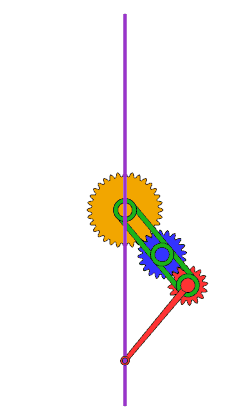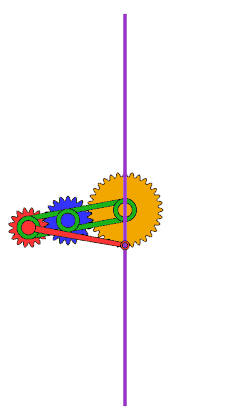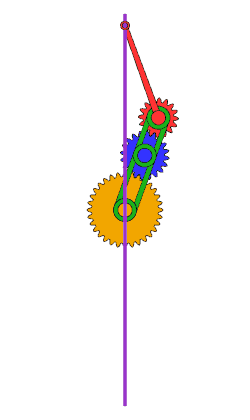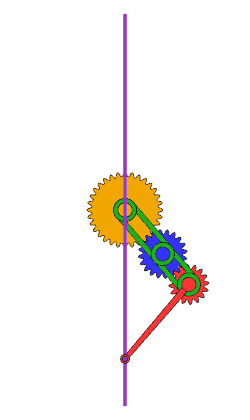
Technical usage

@MichaelFrey: The recently added section on technical usage anachronistically suggests that the hypocycloid Straight-line Mechanism is historically related to the Tusi couple. Although they may be geometrically equivalent, the material presented suggests that the nineteenth-century inventors were credited for creating a new mechanism for producing reciprocating straight line motion rather than applying al-Tusi's discovery.

I don't feel this discussion belongs in this article on the Tusi couple, perhaps it could be added to the article Straight line mechanism under the heading Hypocycloid straight-line mechanism (Tusi couple). If retained in this article, the discussion should make it clear that we have a nineteenth-century discovery of an equivalent mechanism to the Tusi couple for which no historical connection has been demonstrated. --SteveMcCluskey (talk) 18:41, 5 January 2017 (UTC)[reply]

Receiving no further comment, I edited the section on mechanisms to unify the various discussions, clarify their independence from the Tusi couple, and reduce their size. Editors may wish to rescue the detailed discussion from the article history and put it in Straight line mechanism in some form.--SteveMcCluskey (talk) 04:36, 14 January 2017 (UTC)[reply]

"Goodman couple" is 500 year old Cardano invention

That reference seems to be the only mention of this on the Internet, and it says nothing about it being invented by Goodman, nor being named after Goodman, only that he made a video of some gears. Is there any info to the contrary?

The "Goodman couple" appears to be at least 5 centuries old as it was described by Cardano in the 16th century, now known as the "Cardan straight line mechanism." I personally suspect it can be traced to ancient Greece, and will attempt to prove it.

Skintigh (talk) 06:08, 10 February 2023 (UTC)[reply]