Talk:Gaussian beam
| This article is rated B-class on Wikipedia's content assessment scale. It is of interest to the following WikiProjects: | |||||||||||
| |||||||||||
Paraxial approximation
[edit]As far as I understand it, the derivation of the Gaussian beam as a solution to the wave equation relies crucially on the assumption that the rays comprising the beam (meaning the wavefront normals) are paraxial rays, which means by definition that θ is much smaller than 1, which likewise means that θ is very nearly equal to its tangent. If this condition is not true, then the entire solution is invalid, and the beam is not Gaussian. In other words, a Gaussian beam always consists of paraxial rays.
Is that not correct? If not, please explain the issue.
Thanks.
-- Metacomet 07:08, 17 February 2006 (UTC)
Yes, I think you're probably right. I had taken my interpretation from Yariv's book, which says
- This last result is a rigorous manifestation of wave diffraction according to which a wave that is confined in the transverse direction to an aperture of radius wo will spread (diffract) in the far field...according to [the equation above].
I took this to imply that the tan θ form was accurate even in the regime where the paraxial approximation fails, but it's not clear that this is the case. Siegman uses the linear form, and also shows explicitly that the gaussian beam model fails for cases where the divergence is greater than about 30°. He gives references to papers that give higher order corrections to Gaussian beam propagation theory. Note that θ need not be much smaller than 1 rad according to Siegman's analysis, though. --Srleffler 23:22, 17 February 2006 (UTC)
Conventions
[edit]I note that there is an implicitly assumed $\exp(+i \omega t)$ time convention on all the presented forms of the different beam profiles. This stems from the $\exp(-i k z)$-like terms for the spatial parts.
I think the article is in need of a note to explicitly clarify this point - perhaps linking to the concept of time conventions and time harmonic representations of fields in general.
Edward J. Grace 12:57, 20 April 2009 (UTC) —Preceding unsigned comment added by Graceej (talk • contribs)
- I Agree.
- The typical convention, which can be seen in many books, is to have a phase e^ikx-iwt (Physics), while in engineering books - e^-jkx+jwt. Writing e^-ikx may cause confusion. If there will be no objections, I will add this point, and try to keep the convention in this article the same as most other Wikipedia articles in optics and in physics.
- Note that you are replying to a message that is over nine years old. The factor does not appear in the expressions because the expressions give the electric field amplitude, not the whole electric field.--Srleffler (talk) 02:46, 16 May 2018 (UTC)
- Thank you. I'm aware of the time passed. The point is not the factor, but rather the . Yuvalr (talk) 20:13, 28 May 2018 (UTC)
Notation
[edit]I reverted Metacomet's reversion of Pmg's notation change. I don't intend to start an edit war on this, but I do prefer the small-w notation (as apparently does Pmg), and the lowercase notation is used in the figure on this page. This article used this notation until about six weeks ago, when Metacomet changed it from w to W, while doing a major upgrade to the contents of the page. I objected at the time, but he disagreed and I didn't pursue the matter. I understand his/her concern that w is (in some fonts) too easily confused with ω. The lowercase notation is used in Yariv's book and, I think, in Siegman's. The uppercase is apparently used in Saleh and Teich. Obviously we need to arrive at some consensus on notation. I'm not sure how best to do that in this case. Anybody have any thoughts on this issue? The confusion between beam size and angular frequency is not a big problem. I actually used a lower-case omega (which in my handwriting is distinct from a lower-case w) for beam size for years without any problem. Context makes it clear enough.--Srleffler 01:59, 16 March 2006 (UTC)
- First of all, I don't see what the big deal is about using uppercase W versus lowercase w. It's the same letter in the same alphabet. It's not like we are debating whether to use f or ν (that's Greek nu, not Roman vee) to represent frequency, which are two different letters from two different alphabets.
- That being said, I would prefer to use the uppercase W instead of the lowercase w for the reason that Srleffler has already attributed to me: namely, lowercase w does appear quite similar to the Greek letter ω, particularly in the font that shows up in LaTeX equations on WP. Now, you have argued that you have no difficulty telling them apart based on context. I have little difficulty figuring it out either, because I have a lot of experience in physics and electrical engineering. I am not concerned, however, for my own sake; and given Srleffler's extensive knowledge of this topic and other optics-related concepts, I am really not concerned about his ability to tell the difference either. I am concerned about people who are learning this material for the first time, who may or may not readily distinguish between the Greek ω and the Roman w.
- I encounter undergraduate students almost every day who do not know the difference between these two letters for the simple reason that they have not had much exposure to the Greek alphabet and in particular to angular frequency, which is often represented by ω. Then, after they finally start to get the hang of it, suddenly someone reverses course on them and starts using w in the context of optics, which is of course related to electromagnetic waves, which are of course characterized by angular frequency. And now they see w and think its ω!
- Again, it's not a problem for me. I can recognize it quite readily by context and even from the font, because I am aware of this pitfall. But ask a freshman or sophomore who is just starting out in physics or engineering, and you will find that they typically haven't had enough exposure yet to know the difference.
- Finally, I do not find it very compelling to argue that we should use w because that is what the image accompanying the article uses. That is clearly putting the cart before the horse. If it makes sense to use W, then we should use W, and we can arrange to have the image revised. If, on the other hand, it makes sense to use w, then we should use w. But it has nothing to do with the fact that the image currently shows it one way or the other.
- Now, if someone would like to provide a valid reason why lowercase w makes more sense than uppercase W, I would be more than willing to listen to that argument and give it consideration.
- When I made the image, I went with w simply because the reference I used (Koechner) uses that notation, as do my old course notes. If there's a consense to change to W I can easily change the image to match, though I have no strong preference either way. --Bob Mellish 05:41, 16 March 2006 (UTC)
- Lowercase w seems to be the more common notation--Srleffler 12:26, 16 March 2006 (UTC)
- Question for Srleffler (or others): Do you think that using uppercase W will cause any great confusion to the typical WP reader even though the "more common notation" is lowercase w? In other words, do you think that people will have much difficulty realizing that uppercase W represents the same thing that they might have (or might not have) seen in a reference book on this topic? I think you can tell what my opinion is, but please think about it and answer this question honestly. -- Metacomet 14:46, 16 March 2006 (UTC)
- BTW, your statement that lowercase is more common may or may not be true. You are correct to note that Saleh and Teich use uppercase W. So from what we know on this page, there are two books that use lowercase, and one book that uses uppercase. Although that is a two-to-one margin, it is not exactly a huge sample size. And if I happen to find just one more reference that uses uppercase W, then the picture changes significantly. -- Metacomet 14:49, 16 March 2006 (UTC)
- I, as well, do not care whether it is W or w, but i want it to be consistant between the cross referenced articles that refer to this quantity. In addition to this article that includes at least [Complex_beam_parameter] and [Ray_transfer_matrix_analysis], but there might be others. So if you/we revert it back to W(z), make sure to change these articles as well. --Pmg 06:57, 17 March 2006 (UTC)
L'Hôpital's rule
[edit]Jones, I don't see why you insist on showing the first step in the derivation of the peak intensity. It really doesn't seem all that important. In fact, I think that mentioning that the derivation is done using l'Hôpital's rule is already too much information. Notwithstanding the fact that some other articles show derivations, Wikipedia is not a textbook or an instruction manual. Derivations are really not appropriate content, especially when the derviation is not absolutely central to the subject of the article.--Srleffler 00:43, 23 October 2006 (UTC)
130.184.83.26 03:51, 27 March 2007 (UTC) Edited the Siegman citation from Chapter 6 (Laser Pumping and population Inversion) to the more applicable Chapter 16 (wave optics and gaussian beams).
Characteristic Impedance
[edit]The value of characteristic impedance is wrong, and reflects a common mistake in both undergraduate and graduate texts where the characteristic impedance of free space is stated to be EXACTLY 120 pi and therefore approximately 377. It is only exactly 120 pi if you assert that the speed of light equals 3x10^8.
characteristic impedance (free space) = Sqrt[(mu_0/eps_0)] = mu_0*speed_of_light_in_vacuum
If we assume that c = 3x10^8, then this becomes:
4*pi*10^-7 3*10^-8 = 120 pi
But, since c = 299792458
4*pi*10^-7 299792458 != 120 pi ~ 120 pi ~ 377
65.202.227.181 (talk)mjd 2008-04-18-17:08EDT —Preceding comment was added at 21:07, 17 April 2008 (UTC)
Field equation for Hermite-Gaussian beams
[edit]The formula giving the laser field makes heavy use of the complex beam parameter q=z + i zr:
I suppose that it is to conform with the subsection on q:
a gaussian field with one transverse dimension is proportional to
- .
I wouldn't object if all the terms had an obvious physical meaning, but I find the term
particularly cryptic. For instance q0/q0* is just -1! In fact this term (the 2n/4 exponent part) is the Gouy phase for high-order HG beams, it is just a phase-shift of n*ArcTan(z/zr).
The preceding term Sqrt(q0/q) has the advantage to comply with what was said in the "complex beam parameter" section, but physically it mixes two functions: it adapts the normalizing factor 1/Sqrt(n!2^n w0) to the variation of w with z, and it adds a Gouy phase-shift, except that this phase-shift is half what is need: Arg(Sqrt(q0/q))->Pi/4 at infinity instead of Pi/2 (that's why I had to add 1/4 to the exponent of the next term, which, until recently, was n/2 ).
Other forms exist for this formula, for instance, staying close to the above form:
or even, totally suppressing q:
Now each term has its function:
- 1+2: normalization
- 3: Hermite transverse mode structure
- 4: global Gaussian profile
- 5: spherical wave-surfaces curvature
- 6: Gouy phase
May be the two forms should be given, one for people using q, one for people preferring w(z) ?
BTW, the Laguerre-Gaussian formula just below has also to be checked: one should at least define psi and psi0.
--Bruvi (talk) 16:33, 24 June 2008 (UTC)
- I think it would be good to use a simple, intuitive form, and that having equations in terms of both q and w(z) is a good idea. The complex beam parameter is extremely useful in beam calculations. More important, though: we should have an equation that is supported by a citation to a reference work. That will help us avoid further errors such as the one you found.
- Does your version of the Hermite-Gaussian field equation actually take the right form for n=0? It's not clear to me that it does, but I'm too tired right now to work it out.--Srleffler (talk) 04:04, 25 June 2008 (UTC)
Higher Order Modes
[edit]I think that transverse modes should be distinguished from longitudinal modes (which also have varying order). For one thing, the modes which become degenerate at the hemispherical limit or the confocal limit do not belong to the same longitudinal order.
Additionally, I propose a generalization to the phrasing about the transverse modes. Shouldn't the article mention the completeness of the orthogonal functions? Shouldn't it point out that the Gaussian beam represents the zeroth order element of all of the complete sets of orthogonal functions (i.e. constant)? In other words, the Gaussian beam can be regarded as the lowest order element in an expansion over an arbitrary set of complete, (and for convenience,) orthogonal functions. I don't think an artificial separation should be drawn between the Gaussian beam and the other elements of such an expansion. Sorry, I don't have time to formalize this into proper form for presentation on the page, but I wanted to share these ideas anyway.
128.223.23.104 (talk) 20:44, 13 November 2008 (UTC)Justin Hannigan
- This article has really started to overlap with Transverse mode. It may be time to look at the best division of material between the articles.
- There is one reason why Gaussian beams are artificially distinguished from the other possible basis modes: they have the lowest M² of any mode, and are commonly used as a model for an "ideal" laser beam. For this reason, we tend to choose orthogonal basis sets that have the Gaussian beam as the zeroth-order element. There are lots of orthogonal sets that do not contain the Gaussian beam, but they are much less useful for modeling laser radiation.--Srleffler (talk) 05:44, 14 November 2008 (UTC)
- Thanks. I somehow overlooked that page.
- Yes, the Gaussian beam has some nice properties that distinguish it from higher-order transverse modes. However, in the context of expanding the diffracted light that passes through an aperture with Gaussian transfer function in the transverse plane (i.e. a Gaussian aperture) the Gaussian beam will be the lowest order member of each expansion. (I mention diffraction because this is one of the ways to derive the properties of the Gaussian beam.) The lowest order member of each complete set of polynomials in the 2-d plane is a constant, so the resulting lowest order beam (regardless of which set of complete polynomials I pick) will be the Gaussian beam. The existence of Bessel beams or any other type of beam which can be produced by diffracting through a different kind of aperture only introduces a different envelope function. The generalized intensity distribution may still be described by a set of polynomials which are elements of a complete set in the 2-d plane and which, in turn, are all modified by the distinguishing envelope function. The lowest order beam will always just be the envelope function itself (i.e. constant times the envelope). Please correct me if I made a mistake here, but that is consistent with my knowledge of both orthogonal functions and optics. For these reasons, I view each transverse mode as simply a component in an expansion -- analogously to a component in a Fourier series. Yes, the fundamental is important -- but I don't think it should be artificially separated from the rest of the series.
- Anyway, I generally appreciate the quality of physics articles on Wikipedia and just wanted to give my perspective on this small point. I agree that the bulk of discussion of transverse modes belongs on its own page. I just thought the phrasing that is in this article suggested a more rigid separation between the lowest order mode and the transverse modes than I would choose to portray. 128.223.32.10 (talk) 19:46, 14 November 2008 (UTC) Justin Hannigan
- It may come down to practical reasons, rather than fundamental principle. It is common in introductory courses on laser physics to spend a lot of time on the mathematics of Gaussian beam propagation, and relatively little on higher order modes. This reflects the fact that the TEM0,0 mode is an important special case, that is a good representation of many real lasers' output. It also reflects the fact that the mathematics used for propagation of Gaussian beams can easily be extended to treat the other modes. Gaussian beams are such an important special case in practice, that we need to have an article about them specifically.
- About basis sets: I mostly agree with what you wrote above, but I was thinking more generally. For example, the set of plane waves travelling in every possible direction is a complete basis set for electromagnetic fields. You can describe any light wave in free space as a superposition of plane waves. This basis set obviously does not have the Gaussian beam as its lowest-order mode. As far as I can see, this should still work on a beam that has passed through an aperture. Basis sets that have a Gaussian function as their lowest-order member are a more natural and usually more useful basis, but this is merely a choice we make for convenience.--Srleffler (talk) 09:25, 15 November 2008 (UTC)
- On further reading I don't have much disagreement with the phrasing. But it isn't mentioned that the polynomials must form a complete set. And I would suggest some phrasing to say that Hermite, Laguerre and Ince polynomials are only 3 possible sets of functions, not necessarily the only ones.
- It will work. It's just that the set of plane waves following transmission through a Gaussian aperture or emitted from a resonator with spherical mirrors will sum to produce a Gaussian envelope -- possibly with other structure. But I don't find this surprising since boundary conditions generally reduce the symmetry implied by free space.
- It's not the Gaussian envelope, itself, that is a member of the transverse basis. The Hermite, Laguerre, Ince or any other complete set of functions forms a basis. I.e. I could expand any 2-d scalar function in terms of Hermite polynomials. 128.223.23.104 (talk) 20:17, 18 November 2008 (UTC) Justin Hannigan
Laguerre-Gaussian modes
[edit]I would like to raise a concern regarding the figure of the high order LG modes. I am sorry but it is wrong: LG modes are cylindrically symmetric, and those in the figure are clearly not. Pure LG modes have a number of dark rings given by the number p, while the orbital angular momentum number $\ell$ affects the phase, so its effect is more clearly visible if we plot the argument of the amplitude. The only difference in intensity is the radius of maximum intensity, which grows as $\sqrt{\ell}$. --ziofil 23.31 3 November 2012 —Preceding undated comment added 03:31, 4 November 2012 (UTC)
- The modes as plotted are superpositions of and modes; i.e., the phase factor is replaced by . This is done (I assume) because these are the intensity patterns you expect to see when working with linearly polarized light. I put a note in the figure caption, but in the longer term someone may wish to add a note in the text of the article, or else redo the diagram. Zueignung (talk) 06:49, 4 November 2012 (UTC)
- Independently of what one sees while working with polarisers, the equations in the text are not vectorial equations, they are solutions of the scalar wave equation, i.e. polarisation is never considered. It doesn't make much sense in my opinion to show an equation and then show intensity plots of something different. I have produced a new version of the same graphics grid without superpositions of and . How can I share it for consideration as a replacement? User:ziofil 23:26, 10 November 2012 (UTC)
- On the left-hand side of the page, under "toolbox" there is a link called "Upload file". This will walk you through uploading the image. Zueignung (talk) 02:01, 11 November 2012 (UTC)
- Done, it's at the address LG modes. User:ziofil 04:06, 11 November 2012 (UTC)
- I like the new image, and have added it to the article. I notice, though, that the file is in "pdf" format. Would it be possible for you to export the image in a straight image format, and upload it again (with a new filename)? PNG format would be best, but JPEG would also be fine. These formats are more portable, and less dependent on browser plugins.--Srleffler (talk) 23:13, 11 November 2012 (UTC)
- Done, it's at the address LG modes. User:ziofil 04:06, 11 November 2012 (UTC)
- On the left-hand side of the page, under "toolbox" there is a link called "Upload file". This will walk you through uploading the image. Zueignung (talk) 02:01, 11 November 2012 (UTC)
- Independently of what one sees while working with polarisers, the equations in the text are not vectorial equations, they are solutions of the scalar wave equation, i.e. polarisation is never considered. It doesn't make much sense in my opinion to show an equation and then show intensity plots of something different. I have produced a new version of the same graphics grid without superpositions of and . How can I share it for consideration as a replacement? User:ziofil 23:26, 10 November 2012 (UTC)
- Here it is in jpg: LG modes jpg User:ziofil 06:07, 15 November 2012 (UTC) — Preceding unsigned comment added by 173.33.115.183 (talk)
- Thanks.--Srleffler (talk) 07:01, 16 November 2012 (UTC)
- Here it is in jpg: LG modes jpg User:ziofil 06:07, 15 November 2012 (UTC) — Preceding unsigned comment added by 173.33.115.183 (talk)
Missing Info
[edit]There is one very important thing that is not described in this article. Suppose a laser is emitting a laser beam and there are no other optical elements in its way (no lenses, polarizers, etc.). This article implies that the emitted beam will take the form of a Gaussian beam (or at least we can assume it). My question is this: WHY does a beam emitted by a laser has to have the beam waist (i.e., why must it converge to the beam waist and then diverge beyond it)?--193.219.52.43 (talk) 11:38, 20 November 2009 (UTC)
- A laser beam doesn't have to have Gaussian form, but all beams will have a waist. Beams diverge as they propagate away from the waist due to diffraction. The narrower the waist, the faster the beam diverges. This behaviour is fundamental, part of the nature of light. It's not limited to lasers, but non-laser light sources typically diverge faster than diffraction requires, so you don't see the effect of diffraction in everyday life.
- The Gaussian form is important because it has the lowest possible ratio of divergence to waist size. This isn't obvious, but can be proven mathematically. Many lasers do produce Gaussian beams. It's common only because we design lasers for this desirable characteristic. More powerful lasers tend not to produce pure Gaussian beams. One sacrifices beam quality for higher power.--Srleffler (talk) 05:28, 21 November 2009 (UTC)
- You're right, the beam waist can be located inside the laser cavity and be re-imaged by optical elements (collimator...). When a laser beam is re-imaged by optical elements, the waist (w0) is modified as well as the position of the waist w0. —Preceding unsigned comment added by Finglas (talk • contribs) 15:46, 11 September 2010 (UTC)
Could someone add the information that the beam radius is the radius at 1/e^2 of the maximum intensity ? This is not defined in the article, also, the spot size should be defined (some define it as the width at half the intensity, some as the width at 1/e^2)Finglas (talk) 15:54, 11 September 2010 (UTC)
- The fact that w is the radius at 1/e2 is already mentioned, in the section "Mathematical form". I added a link to Beam diameter, which covers different definitions of beam width in some detail. --Srleffler (talk) 19:56, 11 September 2010 (UTC)
- Indeed, it's a bit not clear to me about beam radius definitions. Now w(z) is defined as "the radius at which the field amplitudes fall to 1/e of their axial values". Is it really so ? I think field amplitude should drop in axial values as well as at 1/e distance from axis proportionally with increasing z coordinate. I.E. no matter at what distance (z) from beam waist you will measure beam intensity - you will get the same Gaussian profile. Thus if w(z) defined in such way - why it should be dependent on z at all ? I see no reason. So I'm second which opts for radius w(z) definition to be like "the radius at which the field amplitudes fall to 1/e of their maximum intensity at beam waist (w0)" — Preceding unsigned comment added by 5.20.182.75 (talk) 15:49, 4 January 2021 (UTC)
- Yes, it's really so. The beam expands as it propagates away from the waist in z, so obviously w(z) is a function of z. --Srleffler (talk) 04:52, 5 January 2021 (UTC)
It's not so obvious to me. It depends on HOW you define function w(). As it is now "the radius at which the field amplitudes fall to 1/e of their axial values". Means that at EVERY beam point z=0..x which is at some radial distance r > 0 from AXIAL position (r=0), field strength drops to 1/e, thus forming Gaussian profile at each z coordinate. I.E., field amplitude falling happens everywhere along beam axis. I find this definition inconsistent, unless I incorrectly interpret a term "axial values". Can you please clarify what "axial value" exactly means ? (p.s. I would understand if field strength dropping would be normalized with respect to initial field value at beam axis at beam waist,- position z=0 - but current definition of w(z) does not includes beam waist !) — Preceding unsigned comment added by 5.20.182.75 (talk) 09:21, 5 January 2021 (UTC)
- I'm not sure what you're having trouble understanding here. First, I want to make sure we're using the same definitions: z is the distance along the axis of the beam (the direction in which the beam is traveling). r is a distance perpendicular to that axis. For a Gaussian beam, you can measure the electric field amplitude as a function of r at any point along z and find that the amplitude follows a Gaussian curve: . The value of w depends where you are along z though. Near the waist the beam is narrow, so w is small. Far from the waist the beam is wider so w is larger. Since the value of w varies with z you can write it as a function of z: w(z). The article gives the formula for this function.
- At any given value of z, the field at r = w(z) is 1/e times the field at r=0:
- ( is some function that depends only on z)
- The "axial value" you're asking about is just the value of the field on-axis at any given z: E(0,z).
- I don't know what you mean by "current definition of w(z) does not includes beam waist". I can't find any way to interpret this statement that would make it true. The definition of w(z) certainly does include the beam waist. The value of w(z) is smallest for z = 0.--Srleffler (talk) 23:09, 9 January 2021 (UTC)
Explanation of Gouy phase in error.
[edit]I propose to remove the following wording, because I don't think it is accurate: "Physically, this shift is the result of the uncertainty principle.[2] The transverse confinement of the beam at the focus necessarily implies an increase in the uncertainty in its transverse momentum, which leads to a change in phase of the beam."
Unfortunately I don't have a copy of Mandel & Wolf handy (but am willing to go to the library if this is contested). However I don't believe that the uncertainty principle could possible be cited as a cause of the Gouy phase for the simple reason that it is predicted simply on the basis of classical electromagnetic theory (not dependent on plancks constant). It may well be that one can demonstrate a consistency with the uncertainty principle in regards to some consequence of the Gouy phase, but if so that's just a reaffirmation of the correspondence principle. The explanation given doesn't shed any light on the origin of the Gouy phase. The correct explanation of the Gouy phase is that it is a near-field phenomenon (and almost impossible to directly observe!) in order for Maxwells equations to be satisfied in regions where the electric and magnetic fields are rapidly changing.
Also, the section does not correctly explain that this is an additional phase on top of the usual k*z (on-axis, where x=y=0). Also, the Gouy phase which appears in the equation under "Mathematical form" describing the gaussian beam, is the one symbol that is not identified beneath the equation, which it should be with a reference to the section on the Gouy phase.
But in summary, I think the wording regarding the Gouy phase is incorrect or at least unhelpful to understanding anything about it. I intend to rewrite that portion with no reference to the uncertainty principle. Interferometrist (talk) 12:12, 24 October 2010 (UTC)
- Hold on. A reference to a reliable source trumps an editor's opinion as to what is correct. If you want to argue against that explanation, you need to at least read the cited reference. To remove the explanation from the article, you are going to need to convince the rest of us that you are right. You might need an equally reliable source that explicitly refutes the existing reference. Note that the reference cited is not Mandel and Wolf, but rather:
- Feng, Simin; Winful, Herbert G. (2001). "Physical origin of the Gouy phase shift". Optics Letters 26 (8): 485.
- If you want to add a classical explanation of the Guoy phase shift, go ahead. It might make the most sense to explain the classical understanding of the phenomenon first, and then mention that it can also be understood as a manifestation of the uncertainty principle.
- I think you are being too narrow in your understanding of what a quantum phenomeon is. Ultimately, the world is (to the extent of our current knowledge) a quantum world, not a classical one; classical physics is an approximation to the quantum truth. If a phenomenon can be described in a simple way as a manifestation of quantum physics, it is useful to provide that explanation, even if there is also a good classical explanation for the phenomenon. Of course we should provide the classical explanation too. --Srleffler (talk) 15:42, 24 October 2010 (UTC)
- "A reference to a reliable source trumps an editor's opinion as to what is correct." Well of course you're absolutely right! That's why I put the matter on the talk page first. But even if the statement is unquestionably true, including it in Wikipedia (especially if no one can understand it as presented; I sure didn't!) is a separate issue. But not one I wanted to make a big deal about. And indeed I will lookup the reference (that you have correctly pointed me to) and perhaps get back to you (I gather you watch this page). Just out of curiousity, are you the one who added that paragraph, and if so, can you say that you understand the explanation? (Please don't take that the wrong way: I'm not trying to put you on the spot, and it is perfectly acceptable for editors to include facts they don't personally understand as long as they have transcribed them correctly from a reputable source, which Optics Letters surely is).
- Thanks for dealing with my other two concerns in relation to the Gouy phase. Just what I had intended :-) Interferometrist (talk) 19:23, 24 October 2010 (UTC)
- I don't believe I added that statement to the article. I don't fully understand it, but it doesn't surprise me. In general, diffraction of light is related to the uncertainty principle. --Srleffler (talk) 04:30, 26 October 2010 (UTC)
- Alright, but again that has to do with the "weak form" (for lack of a better term) of the uncertaintly principle, not the one that has to do with quantum mechanics. I understand you didn't have time to answer the discussion I added below (and you don't really need to though I'm interested in any disagreement or reinterpretation you might supply). But I just wanted to point out that if you really DO think the uncertainty principle should be cited as a CAUSE of the Gouy phase, then just saying that isn't very useful. Rather you'd need to mention the integral given in eq (4) which supplies that connection. Otherwise it doesn't really say much beyond "being consistent with the uncertainty principle" which isn't notable. Again, I think the article would be better (less likely to mislead someone) by just removing the sentence. I think that the title of the paper ("Physical origin of...") is rather pretentious and shouldn't give the observation in the paper more weight than justified by its contents. I would simply say that the Gouy phase is one phase factor needed in the equation for the electric field at the top of the page in order for it to satisfy Maxwell's equations, and is a near-field phenomenon, if you need it further "explained." Interferometrist (talk) 16:53, 26 October 2010 (UTC)
(Previously added:)
Alright, it's a matter of little importance regarding Wikipedia but still of interest to me, so I went ahead and looked up the paper and read through it enough to say that I understand the progression of his derivation. My conclusion is that there is probably a valid argument but that treating this matter in terms of statistical distribution of transverse momenta (eq. 2 - 4) is a totally convoluted way of going about deriving something that requires no "statistical" consideration at all. I can hardly think of any case in which I would voluntarily introduce a statistical argument into a deterministic problem in order to make it "easier" in some sense. Note that there is no discussion of or even reference to photons, where statistics would naturally enter into an optics problem, and no dependence on h (as I said, it IS a classical phenomenon, as we all agree). So (as I first conjectured) the possibility of presenting a statistical argument which agrees with the result that would be obtained in the classsical case is not surprising since that would be required by the correspondence principle, if one is to adopt their notion (which I don't like and don't think is very meaningful) that the (classical) angular spectrum decomposition of a beam can be viewed as a statistical combination of transverse momenta (in their words: "A finite beam, however, will have a spread in transverse momentum because it is made up of an angular spectrum of plane waves obtainable by means of a Fourier transform."). What's more I don't really understand eq. (3) which appears to be trying to use the k_x, k_y to have to do with uncertainties rather than their usual meaning of a propagation vector (as in eq 1) and why they can just define k_z-bar as if it's something relevant and then use it according to the little formula in the text (with footnote 13) which applies to the ORDINARY not "effective" z-component of the wave vector. In short, if this argument is valid (not just leading to a correct result) and is explained correctly, then it is one of the most convoluted explanations I can imagine.
And further, it has nothing to do with the uncertaintly principle as I normally understand it. The only way one could say that something like the "weak form of the uncertaintly principle" (I explain below) applies, is in the above strained identification of the transverse components of the angular spectrum being "uncertainties" which you can treat statistically. They don't even mention the uncertainty principle by name until the bottom of the last page. And this is not the quantum-mechanical uncertainty principle in which h is a key parameter (such as delta E * delta t > h-bar) but a classical version whereby one can explain a classical diffraction pattern, for instance, in terms of Aperture * Divergence > lambda, what I call the "weak form" which isn't dependent on any quantum mechanics involving photons or other quanta, just wave theory.
As far as Wikipedia goes, I'll let you decide whether to leave the statement in there or not, but in my opinion it sheds no light for the average reader. I was happy to look this up because this is the sort of thing I read anyway, but I wasn't impressed by this paper. Cheers, Interferometrist (talk) 22:12, 24 October 2010 (UTC)
- I may end up agreeing with you about removing the line once I've read the paper and thought about this some more, but your talk about a "weak form" of the uncertainty principle caught my interest. The paper may have erred in talking in statistical terms, but the angular spectrum of a diffraction-limited Gaussian beam is the same thing as uncertainty in observations of the beam's transverse momentum. If you set up an experiment to measure transverse momentum of individual photons in the beam, the beam's angular spectrum directly determines the spectrum of values you observe. The diffraction-limited relation between aperture and divergence is the same thing as the relation between the uncertainties in the transverse components of photon momentum and position. They are two equivalent ways to express the same underlying physics.
- It's not fundamentally meaningful to assert that an optical phenomenon is not quantum merely because it can be described "just" by wave theory. Light is not a wave. To the extent that light can be considered a wave, photons are waves. It's all the same thing. It doesn't matter whether you think of light as a wave, or as photons, or if you instead decompose the state on some completely abstract basis. If you do the math right, you will get the right answer. (Of course the problem may not be equally easy each way.) The world is quantum all the way up. It doesn't become classical in the classical limit; it's just easier for us to understand when we think of it that way. If a purely classical phenomenon also has a simple quantum explanation, that is a useful and interesting thing to mention.--Srleffler (talk) 02:32, 27 October 2010 (UTC)
- I guess it shouldn't be surprising if we have slightly different appreciations of where classical and quantum physics meet. I had always considered any physical principle not dependent on h (or equivalently, that is found letting h -> 0) to be "classical." And that Maxwell's equations retain their validity in light of quantum mechanics regarding electromagnetic fields, but are only wanting in relation to the transfer of energy or momentum which is quantized. In this particular case, the Gouy phase is predicted from solving the wave equation, classically, whether it be an EM wave or a sound wave, neither requiring nor obtaining greater accuracy through any reference to photons or phonons. I'm sure you don't think that a problem that is solved PERFECTLY using classical physics "needs" a quantum explanation! If the quantum mechanical explanation were easier or actually helped understand the phenomenon, then fine. But the explanation in this paper is really really difficult to even make sense of! I'm not sure it's even valid.
- Have your read it yet? I can't believe you will disagree with me. It is a really difficult argument, and doesn't enhance my (or your, I believe) understanding of the phenomenon. I say ditch it! Interferometrist (talk) 19:54, 27 October 2010 (UTC)
- I agree; the observation in this paper is a curiosity not of sufficiently broad interest to merit being in the body of a page on such a general topic. If the editor who inserted it, who may be one of the authors of the paper, believes this material belongs on Wikipedia, she or he should create a separate page, with an explanation that is sufficiently detailed to be comprehensible without reading the paper. That editor could then insert a link to the new page under the "See also" section of this page.mwregehr (talk) 19:54, 27 October 2010 (UTC)
- Just came to the talk page to question the need for this statement, and found that the issue has already been raised. Offering a 'quantum' explanation (only) suggests that this is not a classical phenomenon, which is misleading - so ditch it. --catslash (talk) 16:53, 27 October 2011 (UTC)
- Given the arguments above, it's fine with me if somebody wants to remove the quantum explanation from the article. I think a good case has been made that this material is not necessary for this article, and doesn't really help the reader understand the phenomenon better.--Srleffler (talk) 23:42, 27 October 2011 (UTC)
- I shall remove it (before somebody offers it as the explanation for stretched wavelength in metal-pipe waveguide (really - see this [1])) --catslash (talk) 15:01, 29 October 2011 (UTC)
History
[edit]Who was the first to calculate Gaussian Beams? Are there any papers on it? I assume it was done first in the 50th or early 60th when Lasers came up. --2001:4CA0:4FFF:1:0:0:0:7A (talk) 09:35, 26 June 2012 (UTC)
Concerns with today's edit
[edit]I have a few concerns with today's edit by Zueignung, which I haven't yet decided how best to address. I thought it would be easier to summarize them here rather than in edit comments.
- There is some redundancy between the second paragraph of the introduction and the new section.
- There is a discrepancy in focus between the new section and the existing lead and "mathematical form" sections. The latter discuss a "Gaussian beam" as a physical thing, whose field amplitude is described by a mathematical equation. The new section talks about Gaussian beams as solutions to an equation, rather than as physical things.
- I don't object to showing a short summary of the mathematical derivation of Gaussian solutions to the wave equation as the new section does, however I'm not sure it belongs right after the introduction. This relates to the "focus" concern, above. I'm not sure yet whether the best fix is to move the section or edit it in place.
- I disagree with the last statement of the new section, "...yields an infinite set of functions known as the Gaussian beams." I would prefer to keep the existing terminology in this article: "Gaussian beam" by itself refers only to the TEM00 mode. Higher-order solutions have distinct family names: Hermite-Gaussian, Laguerre-Gaussian, Ince-Gaussian, etc. I will remove the statement as not supported by any citation, but my objection is as much about organizing material for this article as it is about whether the term is actually used that way somewhere in the literature.
- The section title "Mathematical form of Gaussian beams" violates WP:MOSHEAD.
I do like the treatment of the derivation: just the right level of detail for this article, I think.--Srleffler (talk) 19:01, 19 August 2012 (UTC)
- On looking at this further, I think that moving the derivation later in the article, just before the section on higher-order modes, makes more sense. The existing article introduction already mentions that Gaussian beams are derived by solving the paraxial wave equation. I think it makes the most sense for the article to go directly from the introduction to a description of Gaussian beams and their properties. Wikipedia is not a textbook. The derivation functions nicely as a lead-in to the discussion of higher-order modes, since it answers the question "higher-order modes of what?"--Srleffler (talk) 19:26, 19 August 2012 (UTC)
The reason I used "Gaussian beams" (plural) is because this is the terminology used in Svelto's book, which is what I learned Gaussian optics from. It seems that Siegman does indeed reserve "Gaussian beam" (singular) exclusively for the TEM00 mode and refers to all other modes as Hermite- or Laguerre-Gaussian, so I have no problem with the article following Siegman's convention.
I do not agree with the bit about redundancy. According to WP:LEAD, part of the point of the lead is to summarize the information contained in the body of the article. If you look at a featured article like electron, you will see that the information in the lead is almost entirely redundant with the information in the body of the article, and this is intentional.
The reason I added the derivation right up front is so that one can see where the expression for the field of the TEM00 beam comes from; as things stand now I think it's confusing to have the fundamental assumptions of Gaussian optics come after two sections' worth of detailed exposition about the Gaussian beam. Zueignung (talk) 21:50, 19 August 2012 (UTC)
- I thought about this some more, and I think it isn't so crucial to write down the derivation right away, as long as there are a few sentences at the beginning of the section for the reader to get oriented. As it currently stands, none of the relevant introductory information in the lead has made it into the body of the article. I'll add this. Zueignung (talk) 22:48, 19 August 2012 (UTC)
- I like the changes you've made to the article.--Srleffler (talk) 05:16, 21 August 2012 (UTC)
Versus incoherent beams
[edit]I am getting the sense that coherent beams, and particular single-mode beams, let you focus to a sharper spot than the usual Rayley limit, but I haven't seen it clearly stated. That would explain the desire for laser beams in the first place (versus incoherent beams), but naievely superposition would make me think that coherence wouldn't matter. Looking at Optical_resolution#Lateral_resolution I see whereas at Numerical_aperture#Laser_physics I see and so . Now, . Now, the articles aren't totally clear where the line and are drawn (FWHM vs σ vs ), but whatever the case, the distinctions among incoherent, single-mode, and multi-mode beams should be addressed explicitly. —Ben FrantzDale (talk) 19:00, 6 March 2014 (UTC)
- Yes, a Gaussian beam can be focused to a smaller spot than the Rayleigh limit allows, and in fact it can be shown that a Gaussian beam has the smallest possible product of beam divergence and diameter at its narrowest point. In other words, for a beam of a given diameter, nothing can produce a smaller focused spot than a Gaussian beam.
- Fundamentally, the limit in both cases is diffraction. The Rayleigh criterion is based on the presumption that your optical system's aperture is uniformly illuminated. The beam's transverse profile is a uniformly illuminated circle with hard edges—what laser physicists would call a top hat beam. That profile has much worse diffraction than a Gaussian beam profile does.
- As explained in the article, the width w of a Gaussian beam is the half-width at 1/e2 intensity (HW1/e2M), never FWHM. This is the same as 2σ. Divergence θ is the rate of change in this parameter with distance, in the far field. Outside of mathematical analysis of Gaussian beam propagation, it is common to see the full width (2w) and full angle (2θ) used. It's sometimes not clear whether a quoted divergence is θ or 2θ. --Srleffler (talk) 04:26, 7 March 2014 (UTC)
- Thanks. Do you know of any Wikipedia pages that explicitly state this distinction? Would I be correct to assume that if I took a superposition of the right distribution of modes, the beam shape would approach the Airy pattern I'd expect and the Rayleigh criterion for conventional incoherent light? I've been confused about this distinction for years: the Airy pattern clearly is a strong diffraction pattern a lot like a Bessel beam. It seems like laser people talk about lasers while imaging and illumination people talk about incoherent light and nobody every clearly connects the dots between the two. Thanks! —Ben FrantzDale (talk) 21:06, 12 March 2014 (UTC)
- The articles linked above are clear that the Rayleigh criterion is derived from analysis of the Airy disk, and the latter's article notes that the disk is the diffraction pattern produced by a uniformly illuminated circular aperture.
- Thanks. Do you know of any Wikipedia pages that explicitly state this distinction? Would I be correct to assume that if I took a superposition of the right distribution of modes, the beam shape would approach the Airy pattern I'd expect and the Rayleigh criterion for conventional incoherent light? I've been confused about this distinction for years: the Airy pattern clearly is a strong diffraction pattern a lot like a Bessel beam. It seems like laser people talk about lasers while imaging and illumination people talk about incoherent light and nobody every clearly connects the dots between the two. Thanks! —Ben FrantzDale (talk) 21:06, 12 March 2014 (UTC)
- The Hermite-Gaussian modes (including the Gaussian) form a basis set for electromagnetic radiation. Any beam pattern you can imagine can be formed as a superposition of Hermite-Gaussian modes. --Srleffler (talk) 05:27, 14 March 2014 (UTC)
- Naive questions: Is that to say that a laser beam with a tophat profile will make an Airy disk if you focus it down (considering the plane at which it forms a tophat to look like a uniformly-illuminated circular aperture)? Likewise, are you saying that if I have an incoherent beam and apodize the aperture so that it has a Gaussian intensity profile the Gaussian-beam rules for spot size would apply? (That doesn't sound right to me since (1) it would have many many modes and (2) in a handwavy sense, apodizing seems a lot like stopping down the aperture, so I'd expect a larger diffraction-limited spot size, not a smaller one.) Thanks. —Ben FrantzDale (talk) 14:19, 17 March 2014 (UTC)
- Good questions. Yes, a top-hat beam will make an Airy disk. No, trying to apodize an incoherent beam into a Gaussian beam won't work. Modes are not just intensity profiles, they are profiles of intensity and phase. You have to get both right to make a Gaussian. In fact, except for special cases it is not possible to convert a multimode or spatially incoherent beam into a Gaussian beam. A beam is either single mode or it is not, and if it isn't there is generally no way to make it singlemode except by discarding all power that is in other modes.--Srleffler (talk) 16:29, 17 March 2014 (UTC)
- Naive questions: Is that to say that a laser beam with a tophat profile will make an Airy disk if you focus it down (considering the plane at which it forms a tophat to look like a uniformly-illuminated circular aperture)? Likewise, are you saying that if I have an incoherent beam and apodize the aperture so that it has a Gaussian intensity profile the Gaussian-beam rules for spot size would apply? (That doesn't sound right to me since (1) it would have many many modes and (2) in a handwavy sense, apodizing seems a lot like stopping down the aperture, so I'd expect a larger diffraction-limited spot size, not a smaller one.) Thanks. —Ben FrantzDale (talk) 14:19, 17 March 2014 (UTC)
Error in eqution for Laguerre-Gaussian modes?
[edit]I think there is a small mistake in the current equation for the Laguerre-Gaussian modes: A test with Mathematica (and by hand for the 00-mode) shows that the current solution does not satisfy the wave equation in paraxial approxiamtion for cylindrical coordinates, ie
However, adding a small minus sign such that
seems to fix the problem. If anyone could verify this, we chould change that.
Thank you! 138.232.67.107 (talk) 12:56, 9 May 2014 (UTC)
- Issues like this are often due to a difference in sign convention between different treatments of a subject. If you want to be sure that you are doing the math right, you need to make sure that the same convention is applied throughout your work.
- The article really ought to cite a reference for the formula, so that we can verify it by checking that it agrees with the reference, rather than by doing our own analysis (contrary to policy). --Srleffler (talk) 03:47, 10 May 2014 (UTC)
To-do
[edit]Index of refraction needs to be made consistent through these equations. Right now, I think some of the formulas assume n=1.--Srleffler (talk) 04:38, 29 July 2014 (UTC)
Regarding units of measurement
[edit]Should the units of measurement of physical quantities be included underneath the given formulas? They is confusing without any units being mentioned. Are all units in SI? — Preceding unsigned comment added by 212.117.13.53 (talk) 15:05, 4 October 2015 (UTC)
- No, because in many cases you can use any consistent system of units in a formula and it will work. Units should only be given when the formula is specific to one system of units. If you're going to use one of the formulas, I recommend using SI units, though.--Srleffler (talk) 00:51, 5 October 2015 (UTC)
1/e vs 1/e^2
[edit]At the start of the document, w(z) is mentioned as the 1/e value. In the beam divergence section, to me, w(z) is implied to be the 1/e^2 value. I think that should be clarified. Pjfcarle (talk) 16:33, 8 May 2016 (UTC)
- Intensity is the square of amplitude, so 1/e in amplitude is 1/e2 in intensity.--Srleffler (talk) 18:44, 8 May 2016 (UTC)
Incorrect magnetic field
[edit]As it stands the expression for the magnetic field is simply wrong. One can see by taking the curl of E that the given expression for B is nowhere near correct, with By not being proportional to Ex, and there existing a non-zero Bz component. If approximations were used to obtain this result they should be stated, referenced, and explained in the article. — Preceding unsigned comment added by AwkwardWhale (talk • contribs) 01:55, 17 October 2017 (UTC)
- I don't know for sure, but this may be just a result of using the paraxial approximation, which is mentioned in the article. E and B clearly can't be exactly along x and y; a z component is required off-axis because the wave fronts are curved. --Srleffler (talk) 05:10, 17 October 2017 (UTC)
We should do something about this! I just noticed this as well. (2020)
So I looked into this for a few minutes: First one should use the identity $\curl(e\hat{v})=e\curl(\hat{v})+\grad(e)\cross\hat{v}$. Then assuming k is very large one can drop all x and y derivatives, as the z derivative brings down a k which is much larger... — Preceding unsigned comment added by 213.112.43.180 (talk) 15:33, 11 October 2020 (UTC)
- Don't do any original research. Contributions to the article need to be tied to reliable sources--Srleffler (talk) 19:26, 11 October 2020 (UTC)
I rather give a correct proof than having random incoherent statements in the article @Srleffler. This policy does not make sense as long as one can proof his claims. An elementary proof is the most reliable source. Good way to discourage engagement in Wikipedia. Anyways, as it may be helpful to some, I give the justification I put in my own thesis work:
For a monochromatic wave Faraday's law becomes . As in the scalar approximation for paraxial waves one can apply to find
Assuming sufficiently slowly varying envelopes (SVEA approximation) one can neglect all variations of the envelope in first order. — Preceding unsigned comment added by 130.237.240.81 (talk) 09:01, 15 October 2020 (UTC)
- Even smart and well-educated people make mistakes. We rely on published sources because then we can have confidence that the work was carefully vetted by at least two people.
- I think you're ending up with an inconsistently applied approximation here. When you apply the scalar approximation for paraxial waves and write , you are choosing to ignore the z component of the electric field. The wavefronts are curved; there is actually a z component everywhere off axis. The z component of the magnetic field is similarly ignored in this approximation. Scalar paraxial waves do not exactly obey Maxwell's equations.
- I see that most sources skip over describing the magnetic field by using something like
- without explanation. Perhaps we should do the same.--Srleffler (talk) 21:36, 18 October 2020 (UTC)
- Well I understand that we need verifiability, but I also believe that it is alright to say something that is clearly true even though the literature doesn't say it explicitly, in this case because any student prepared for this subject will already understand that in a plane wave (or approximately, in the portion of a wavefront which is locally "plane") that H_v=E_u / η0 where v, u, and the k vector are mutually perpendicular. I'd hardly call recognition of that fact "OR." Also, I wouldn't call OR the observation that in free space (no sources) the equations for E and H are completely symmetric, so that in a gaussian beam the possible solutions for E and H must be the same (or there are two different types of gaussian beams). And likewise whenever I see P=|E| 2 / η0 that is almost the same as telling me (by division, if you will) that H= E / η0 with them perpendicular (anything else would violate symmetry). I saw as better the previous explanation of I being the result of calculating the Poynting vector, with H specified, than just failing to mention it at all.
- The contradiction about polarization (that H cannot be purely in Y) applies equally to E, given that it was specified that E is in the X direction which is impossible except for a plane wave (or cylindrical wave, if you must), it must be different where the beam is curved from the Z axis and the local Poynting vector is not exactly in the Z direction. If you want to say that is not true, that it IS alright to write E_x because we have assumed the paraxial approximation (to too great a degree!) then it is equally true that H is in the Y direction (again, not really) to that degree of approximation. The best approach to handle this is to say that at any point E is in the U direction, H is in the V direction, and d/dw of the fields' phases (with u,v,w perpendicular) is approximately ω/c (not exactly if you take into account the Gouy phase!) and that if you want to ignore the angle of the wavefronts then U==X and V==Y works. There is nothing different about the magnetic field from the electric field in its mathematical form, so if the previous expression for H was wrong (only claimed to be an approximation!) then so is it for E. I don't know how to go forward after Srleffler went ahead and edited this nicely, but I really think that responding to this {CN} made the article weaker, given that the obvious relation of H to E wouldn't be clear to a lot of less prepared readers. Interferometrist (talk) 23:12, 18 October 2020 (UTC)
- I had no doubt that the equation I deleted was correct. The symmetry between E and H is clear. It came down to simple policy: no source was cited and the sources I had available did not give an expression for H. This discussion is a prime example of why we have this policy on Wikipedia. Here we have two very intelligent people arguing for different expressions for H that they have derived. We could spend hours arguing about this and not end up certain who is right. Fortunately, we don't have to...we solve disputes by relying on what has been published in reliable sources. --Srleffler (talk) 03:34, 19 October 2020 (UTC)
- The contradiction about polarization (that H cannot be purely in Y) applies equally to E, given that it was specified that E is in the X direction which is impossible except for a plane wave (or cylindrical wave, if you must), it must be different where the beam is curved from the Z axis and the local Poynting vector is not exactly in the Z direction. If you want to say that is not true, that it IS alright to write E_x because we have assumed the paraxial approximation (to too great a degree!) then it is equally true that H is in the Y direction (again, not really) to that degree of approximation. The best approach to handle this is to say that at any point E is in the U direction, H is in the V direction, and d/dw of the fields' phases (with u,v,w perpendicular) is approximately ω/c (not exactly if you take into account the Gouy phase!) and that if you want to ignore the angle of the wavefronts then U==X and V==Y works. There is nothing different about the magnetic field from the electric field in its mathematical form, so if the previous expression for H was wrong (only claimed to be an approximation!) then so is it for E. I don't know how to go forward after Srleffler went ahead and edited this nicely, but I really think that responding to this {CN} made the article weaker, given that the obvious relation of H to E wouldn't be clear to a lot of less prepared readers. Interferometrist (talk) 23:12, 18 October 2020 (UTC)
Assuming a TEM polarization is an approximation, yes. But this approximation can be justified by using \nablaE=0 and the SVEA approximation. Doing this one can find that for "paraxial waves" the field components transverse to z are much bigger than the one in the z direction. I never claimed that this is an exact solution, it is not! Exact solutions are messy. They don't just need to solve the Helmholtz equations for all 6 components but also the full Maxwell equations. There are papers about this out there, but the solutions are messy. Much of the literature is only very brief when it comes to discussing the approximate "paraxial wave" solutions and don't worry about polarization. They just treat the fields as scalar. This does not mean we should do this as well! Of course one can make this more rigorous and specify which approximations are made. In the end it comes down do mathematically defining what a "paraxial wave" is. This definition should justify the application of the SVEA approximation for paraxial beams. Indeed one can show a very close and interesting relationship between SVEA and the paraxial approximation. In the theory part of my bachelors thesis I'm doing this. Surely there is literature out there doing similar things, I didn't bother checking though.
Also concerning the expression for P you provided. This power is derived from the integrated intensity, that you get from the Poynting vector if you use the result I proved and your removed from the article. Maybe you shouldn't just discard stuff you don't understand. So you just skipped over the approximation because you didn't find it in whatever book you were looking at, but gave a result which uses this very approximation. This is in some sense quite funny. And no we should not do the same than these lazy text book authors! I think you should roll back the things you removed.
I fully agree with the second paragraph of Interferometrist by the way. His way of interpreting the gaussian beam locally as a plain wave is in accordance with my approximation which uses SVEA. We both arrive at the same results, namely that E and B (I hate that the optics community uses H) are orthogonal.— Preceding unsigned comment added by 213.112.43.180 (talk • contribs) 20:51, 18 October 2020 (UTC)
- As you said, "exact solutions are messy". The point in adopting the scalar, paraxial approximation is to obtain simple, neat solutions that are close enough to correct. You and Interferometrist have both done that, obtaining two different approximate expressions for the magnetic field. Neither one is "wrong". Both give the correct expression for intensity. Interferometrist's has the advantage of being symmetrical with the expression for E.
- By the way, you might want to sign up for a Wikipedia account. It's quick and easy, and you can stay as anonymous as you like. It makes it easier to carry on conversations, though, since an account gives you a consistent "identity".--Srleffler (talk) 03:55, 19 October 2020 (UTC)
@Srleffler We didn't obtain different solutions! I made my proof up there more clear. Plug in the x direction for epsilon and you will find the very apprximation that was in the article before and that Interferometrist is talking about as well. Honestly I don't know how to convince you that you should bring back the form of $B$ in the article. This makes everything much more concise. We can just write that we used the SVEA/paraxial approximation and that these are only approximate results. The equation was extremely helpful when I started studying this topic a few weeks back.
Gouy Phase Figure
[edit]The figure for the discussion of Gouy phase shift compares the phase advance of a plane wave with that of a gaussian beam. It seems to me that the gaussian beam is slowing in the focal region and lagging the plane wave when it should be speeding up and overtaking the plane wave. If this is inddeed incorrect, this could be a sign error in code used to make the figure. — Preceding unsigned comment added by 73.113.140.122 (talk) 15:15, 1 April 2020 (UTC)
According to RP photonics[1] the Gouy phase should be defined with a minus sign, which is different from how it is defined here. This could be a matter of convention though. — Preceding unsigned comment added by 73.113.140.122 (talk) 20:10, 1 April 2020 (UTC)
- Yes, you are absolutely right. As far as sign, you also have to consider that if you use the understood time factor as e^jωt (common in EE) then a negative phase is a delay but if e^-iωt (common in physics) then a negative phase is an advance. But in any case the figure is wrong. And moreover it is wrong geometrically, contradicting the very previous section regarding the curvature vs. z (unless, possibly, if you accept a great aspect ratio exagaration in the "picture". I'm removing it. Interferometrist (talk) 18:51, 3 April 2020 (UTC)
This is a useless article for most readers
[edit]This article is written by experts for experts. It is useless for a general reader who just wants some basic information on what a Guassian Beam is. Isn't that really the first purpose of any encyclopedia article? I am reasonably well educated, and hold a degree in mathematics, but I totally do not understand anything in this article. Shouldn't I reasonably expect to at least have some understanding of the basics of the subject after reading the article? — Preceding unsigned comment added by 2600:1700:5440:d60:2865:aba:1d72:7574 (talk • contribs) 04:45, 3 April 2021 (UTC)
- Not every article on Wikipedia is aimed at a general audience. Articles on more technical topics are often aimed at a narrower audience. We can and should do better here, though. Even technical articles should at least have an introduction that is general-audience friendly.--Srleffler (talk) 06:45, 4 April 2021 (UTC)
- I do sometimes wonder about so many posts complaining about articles. In this case, I at least slightly believe it. Okay: whose amplitude envelope in the transverse plane doesn't seem the most understandable way to explain it. Gah4 (talk) 11:10, 13 April 2021 (UTC)
Intensity of a Gaussian beam around focus at an instant of time, showing two intensity peaks for each wavefront (computer graphics)
[edit]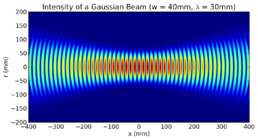
Is it correct not to show wether the image is taken from a photo from an real experiment setup or wether it is a computer graphics. Wouldn’t it be better to put in scopes [computer graphics)? Of course in the data base you’ll see that it is a computer graphics, but to mention it directly under the image is the better practice, isn’t it? — HolgerFiedler (talk) 10:47, 13 October 2017 (UTC)
- I added the word "simulated" to the caption in the article. The image is a graph (not a photo or "computer graphics").--Srleffler (talk) 11:32, 13 October 2017 (UTC)


I do not think this figure should be used. The title does not correspond to the image: It is showing , i.e., the absolute amplitude of the real part of the electric field and not the intensity . If a depiction of the field is desired then the plot should not take the absolute value: . If a depiction of the intensity is desired than the field values should be squared: . The current depiction is neither and is very uncommon and confusing. Also the wavefront is usually defined as a continuous function: the points of equal total phase. Thus, the comment about "two intensity peaks" per wavefront is confusing as well. I have the script ready to generate similar pictures, so if there was something wrong or incomplete with my last attempt, please tell me and I will generate a new version.RealGaussianBeam (talk) 16:57, 10 December 2021 (UTC)
- Listen, it is appreciated when editors go to the trouble to produce figures, but it is best (and least likely to waste their time) when you first discuss the issue right here, just as other things are often discussed before changing them. Especially if you consider that just like YOU did some work to produce your figure, some OTHER editor went through the same trouble previously and it isn't always obvious if what you've done is an actual improvement. There are a number of competent physicists and science communicators watching this discussion who should express state their preference. But I reverted it because I thought the previous figure was overall better, though both had issues.
- Thank you for your reply, I mostly figured out this much, but it was late in the day and mistakes were made. Sorry for the unintended trouble. I did not create the script just for this purpose, it is a part of a python playground that I can give students so that they can familiarize themselves with Gaussian optics. The greatest trouble for me (the confusion notwithstanding) was figuring out what exactly the editor of the last figure wanted to plot. The displayed image was in opposition with the caption and/or title of the figure. RealGaussianBeam (talk) 09:39, 11 December 2021 (UTC)
- I think a plot of field amplitude is more valuable than a plot of intensity envelope which is what I removed. The second graphics you provided above is more like what I'd propose. Now, to indicate -1 to 1 volts/meter you could: A) use a pseudocolor scale with zero being yellow/green; B)Use two different colors for positive and negative field but different shades, as you have above; C) Show the non-complex absolute value or absolute value squared at an instant of time. Your caption about the real part of the phasor of course is the same as saying the amplitude at an instant, and I think that is better since some people reading this won't immediately understand Re{E(r)} but will understand E(t) or |E(t)|. But that just has to do with the caption. Otherwise I'd like others to express their preference between the versions. Interferometrist (talk) 00:34, 11 December 2021 (UTC)

- I used the "magma" color map of matplotlib, as it seems to be onf of the recommended [1] ones to use. (It works for color blind people and does not produce features that are not present in the original data.) RealGaussianBeam (talk) 09:39, 11 December 2021 (UTC)
- Here is the current graphic and caption. The main thing that bothers me about it is that it is for a wavelength of 40mm! I would have called this an article about optics, but I didn't mean microwave optics ;-( Interferometrist (talk) 00:41, 11 December 2021 (UTC)
- Indeed, the wavelength is kind of unfortunate. Especially, if we scale the figure in units of or then the actual wavelength the does not add any useful information. RealGaussianBeam (talk) 09:39, 11 December 2021 (UTC)
@RealGaussianBeam: Listen I think we can come to a quick agreement (but expect more than 2 people to say so!) on where to go with this, and if you're prepared to change a few parameters and upload a new graphic, then do so. Here are my main preferences:
- Use method B, two different colors in varying shade for +E and -E.
- Therefore use something like your current pink plot, but make the pink black which I think is more intuitive. I don't think colorblind readers will have problems, especially as most colorblindness is in only 1 dimension of color perception (plus they're used to using other cues, such as when hitting a traffic light!). I think the most common colorblindness collapses red and green, so instead use perhaps shades of orange and cyan. And it would be more readable on a monochrome monitor (do they still have those?) with the viewer figuring out that adjacent peaks are opposite phase, as with the old graphic.
- Adjust your color maps such that the apparent width of the wavefronts is consistent between +E and -E, which your pink plot doesn't do properly.
- Leave the graphic annotations for Zr and w=sqrt(2)w_0, but place other supporting information in the caption (this is much nicer if a change in wording is later proposed, etc.)
- Specify NO actual units, but use normalized units as you currently do, and specify the NA of the gaussian beam. Make the aspect ratio 1:1 on that basis. Possibly a slightly larger NA (and taller figure), unless you're worried that is no longer "parraxial".
- Instead of the X axis in units of λ which doesn't add any information to the figure (you can see the wavefronts) put it in terms of Z_r.
- For the caption, though this is a separate issue, say that this is the instantaneous electric field at time T, rather than talking about real parts which will confuse some readers and the ones who wouldn't be confused will understand the connection
- Can you make animated graphics? If so, then for extra credit stitch together the same figure produced in 4 or 6 phases :-)
Others watching this page please agree or disagree with any of these points so we can move forward and settle this.Interferometrist (talk) 18:28, 12 December 2021 (UTC)
- I have no objection.--Srleffler (talk) 03:56, 14 December 2021 (UTC)
Animation of LG10 mode
[edit]Researchers at King's College London have created some physically accurate renders of a focused Laguerre-Gaussian 1,0 beam. We would like to insert the following video into the Laguerre-Gaussian modes subsection, and would welcome any suggestions/comments from the community. The 3D animation helps the reader conceptualize the spiralling nature of the wavefronts, how they propagate with time, and highlights how the positive and negative regions of the Laguerre polynomial functions give rise to a double-helix structure.--J.Kingsley-Smith (talk) 12:30, 2 February 2022 (UTC)
— Preceding unsigned comment added by J.Kingsley-Smith (talk • contribs) 12:30, 2 February 2022 (UTC)
- Wow, that is a nice video!Maria Gemmi (talk) 12:34, 2 February 2022 (UTC)
Gaussian beam and TEM00
[edit]I noted in one of the figures of the green laser the image was showing a TEM00. I think this is incorrect, or at least not always correct. Although all TEM00 beams are Gaussians, a Gaussian beam doesn't mean it runs single-mode, i.e. TEM00. For instance you can have a multi-mode beam whose profile is Gaussian as a result of the overlap of higher-order modes. It could be possible that most green laser pointers at 5mW are single-mode, but as the caption states it seems like that every Gaussian is a TEM00, this subtlety would mislead both general readers and experts too.
I would swap showing a TEM00 profile with showing a Gaussian profile. 212.44.19.233 (talk) 15:25, 4 July 2023 (UTC)
- As used in laser physics, "Gaussian beam" and "TEM00 beam" are synonyms. A multimode beam with a Gaussian profile is not a "Gaussian beam". This is not just semantics. Such a beam will not propagate according to the math described in this article. I recall Siegman discussed this in a paper. It might be this one:
- Siegman, A. E. (October 1997). "How to (Maybe) Measure Laser Beam Quality" (PDF). Archived from the original (PDF) on June 4, 2011. Retrieved Feb 8, 2009. Tutorial presentation at the Optical Society of America Annual Meeting, Long Beach, California
- --Srleffler (talk) 03:50, 10 July 2023 (UTC)
Decomposition
[edit]The paragraph beginning "Arbitrary solutions of the paraxial Helmholtz equation can be expressed as combinations of Hermite–Gaussian modes (whose amplitude profiles are separable in x and y..." is way to high level to be introductory paragraph of the content. This should be moved later. Geoffrey.landis (talk) 23:15, 4 March 2024 (UTC)
Moved
[edit]I moved that text into a new section futher down in the article. I'm also going to move the subsection "Gouy Phase" further down, it is not going to be of main interest to a person looking at an encyclopedia article to understand what a Gaussian beam is. Geoffrey.landis (talk) 16:52, 5 March 2024 (UTC)
Incorrect normalization
[edit]The normalization of Laguerre-Gaussian modes is not correct, namely, there is an extra factor in the first formula in section Gaussian_beam#Laguerre-Gaussian modes. The easiest way to see that is to consider the Laguerre-Gaussian beam with , which should reduce to the usual Gaussian mode. According to the formula in the article: with . But then By substitution (, injective on interval ) one can show that the integral in the last line is equal to one:
Overall, the total intensity of the beam for a fixed is equal to , not 1 as one would expect. Also, it means that the formula for the usual Gaussian beam in section Gaussian_beam#Mathematical form is misleading. The formula as it stands in the article: suggests that when is set to 1, the beam is normalized to one (otherwise, one could just include , which is constant, to ), which is not the case. Instead, the total intensity is equal to . JozumBjada (talk) 09:53, 21 May 2024 (UTC)
- I don't follow your last point. From the last formula, just as one ought to expect.
- There has been way too much churn over the years with different editors tweaking the normalization of these functions to match whatever treatment they are used to. There is more than one way to normalize the amplitudes; and different authors vary in what the "amplitude" quantity they calculate actually is. If you remove the in a few months some other bright editor will come along and add it back. I think if you look at the history you'll see it has already been added and removed several times.--Srleffler (talk) 13:39, 24 May 2024 (UTC)
- I understand, but as it is now, the article as a whole uses an inconsistent notion of normalization (and as mentioned above, the formula for the Laguerre-Gaussian beam seems to be not entirely correct). I am used to the normalization that is now used in section Gaussian_beam#Laguerre-Gaussian_modes - that is, the integral of the intensity over the transversal plane should be unity. But given the Laguerre-Gaussian-beam formula in the text, the integral is equal to . If the usual Gaussian-beam formula is normalized such that its amplitude at the origin has a simple form, as you suggest, there could be at least a footnote explaining what is meant by "normalization". (As a side note, all higher-order Laguerre-Gaussian beams are equal to zero at the origin and so this notion of normalization cannot be used.) This way, one could also avoid misunderstanding and overwriting of the formula in the future. JozumBjada (talk) 09:09, 27 May 2024 (UTC)
- Agreed, it would be beneficial if all the equations were normalized the same way. Even better would be if each equation was supported by a reference that uses that normalization, which enables us to simply revert any further change to the formulas. My experience has been that it isn't easy maintaining formulas for electric field and other quantities that have various and/or arbitrary normalization, sign conventions, etc. There is an unending stream of new editors who are familiar with a particular form of the equations and will assume that any other form is wrong, producing continuous churn that is difficult to contain.
- Personally, I like the notation for the Gaussian beam, because it is clear enough not to require further explanation and it makes normalization irrelevant. E simply is whatever it is. As an applied physicist, I would way rather think of E as a physical electric field in volts per metre rather than some arbitrary number normalized to make an integral equal to one, but hiding all the details in leaves everyone free to imagine the equation as normalized however they like.--Srleffler (talk) 16:34, 27 May 2024 (UTC)
- I fully agree. Okay, how about this: I will remove from the Laguerre-Gaussian beam formula, add a reference to [2] (equation (23)), where the correct formula can be found; and add a footnote mentioning that the normalization of the usual Gaussian beam as used in the text is not the same as the one used for the Laguerre-Gaussian beam. Would that be okay? (Btw, I work in quantum optics and the reason why beams are normalized that way (i.e., integral of intensity is equal to one) is because one can then interpret them as a probability distribution. The intensity is then equal to the probability density of detection of a photon at that particular point in space. So it is also physically motivated :) ) JozumBjada (talk) 09:21, 31 May 2024 (UTC)
- That sounds fine.--Srleffler (talk) 07:13, 2 June 2024 (UTC)
- I fully agree. Okay, how about this: I will remove from the Laguerre-Gaussian beam formula, add a reference to [2] (equation (23)), where the correct formula can be found; and add a footnote mentioning that the normalization of the usual Gaussian beam as used in the text is not the same as the one used for the Laguerre-Gaussian beam. Would that be okay? (Btw, I work in quantum optics and the reason why beams are normalized that way (i.e., integral of intensity is equal to one) is because one can then interpret them as a probability distribution. The intensity is then equal to the probability density of detection of a photon at that particular point in space. So it is also physically motivated :) ) JozumBjada (talk) 09:21, 31 May 2024 (UTC)
- I understand, but as it is now, the article as a whole uses an inconsistent notion of normalization (and as mentioned above, the formula for the Laguerre-Gaussian beam seems to be not entirely correct). I am used to the normalization that is now used in section Gaussian_beam#Laguerre-Gaussian_modes - that is, the integral of the intensity over the transversal plane should be unity. But given the Laguerre-Gaussian-beam formula in the text, the integral is equal to . If the usual Gaussian-beam formula is normalized such that its amplitude at the origin has a simple form, as you suggest, there could be at least a footnote explaining what is meant by "normalization". (As a side note, all higher-order Laguerre-Gaussian beams are equal to zero at the origin and so this notion of normalization cannot be used.) This way, one could also avoid misunderstanding and overwriting of the formula in the future. JozumBjada (talk) 09:09, 27 May 2024 (UTC)





![{\displaystyle {u}_{n}(x,z)=\left({\frac {2}{\pi }}\right)^{1/4}\left({\frac {1}{2^{n}n!w_{0}}}\right)^{1/2}\left({\frac {{q}_{0}}{{q}(z)}}\right)^{1/2}\left[{\frac {{q}_{0}}{{q}_{0}^{\ast }}}{\frac {{q}^{\ast }(z)}{{q}(z)}}\right]^{(2n+1)/4}H_{n}\left({\frac {{\sqrt {2}}x}{w(z)}}\right)\exp \left[-i{\frac {kx^{2}}{2{q}(z)}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/05ca96a4cdca57e0f488d9835934ef0b553870df)

![{\displaystyle \left[{\frac {{q}_{0}}{{q}_{0}^{\ast }}}{\frac {{q}^{\ast }(z)}{{q}(z)}}\right]^{(2n+1)/4}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c0a741ad4b45058cdaeb8c530c821cb734846c5f)
![{\displaystyle {u}_{n}(x,z)=\left({\frac {2}{\pi }}\right)^{1/4}\left({\frac {1}{2^{n}n!w(z)}}\right)^{1/2}H_{n}\left({\frac {{\sqrt {2}}x}{w(z)}}\right)\exp \left[-i{\frac {kx^{2}}{2{q}(z)}}\right]\exp \left[i(n+1)\arctan {\frac {z}{z_{R}}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/72c2eb9ed3d5a2524ed04d88f060602e77d98e91)
![{\displaystyle {u}_{n}(x,z)=\left({\frac {2}{\pi }}\right)^{1/4}\left({\frac {1}{2^{n}n!w(z)}}\right)^{1/2}H_{n}\left({\frac {{\sqrt {2}}x}{w(z)}}\right)\exp \left[-{\frac {x^{2}}{w^{2}(z)}}\right]\exp \left[-i{\frac {kx^{2}}{2R(z)}}\right]\exp \left[i(n+1)\arctan {\frac {z}{z_{R}}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8c7042aa592b6dcc0a060daf10cbb40c10182691)

































![{\displaystyle {\begin{aligned}\int _{0}^{\infty }{\frac {4rdr}{w(z)^{2}}}\exp \!\left(\!-{\frac {2r^{2}}{w^{2}(z)}}\right)=&\int _{0}^{\infty }dy\exp \!\left(\!-y\right)=[-e^{-y}]_{0}^{\infty }=0-(-1)=1.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8aefcc16f357369d9ffce4d7f81d8d738d1125f8)







